Growing Triangles, Growing Skills

"You know what I noticed, Malke?" says one of the kids during this week's math groups in my daughter's 3rd/4th grade class, "Everything you've done with us is about triangles."

You know what I've noticed?  I've noticed that making math with the kids in my daughter's 3rd/4th grade classroom once a week is giving me empathy for learning how to teach something brand new.  I'm thinking specifically of the teachers who attend my Math in Your Feet workshops to learn how the program works -- it's not lost on me how much newness there is to manage even in simply getting kids up to learn and think with their bodies, not to mention learning math in a completely new way.

The newness for me is the fact that I am not teaching dance. And, even though I am focusing mostly on having kids explore numbers and number patterns visually, which seems easy enough, the newness is in orchestrating the experience so that we are not just noticing visual patterns but that we also move those observations forward with analyzing the number patterns we see. It's a fun and engrossing challenge, but I'm glad I have four groups to work with because it gives me multiple opportunities to smooth out how I present and sequence the lesson. 

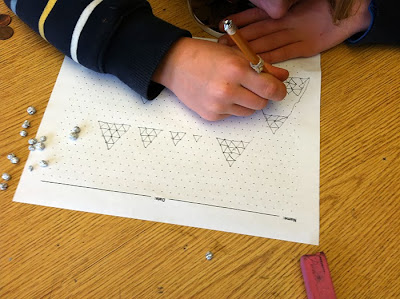
Today we investigated how triangles grow. We got out the pennies and started building.  "You know what I see?" said one girl, "Each new triangle has the old one inside it."

Then we started recording our observations on dot paper, one triangle at a time, including how many pennies it took to make each triangle.

Every minute or two I'd check in with them and get the consensus about the number of pennies in each triangle.  Once most kids got to the fifth triangle we put down our pencils and pennies and started looking for patterns.

During construction/hands-on time, many kids noticed while they worked that each new triangle was built by adding on a new row, and that the new row was one more penny than the row before.  The picture above shows quite clearly the pattern we found in the growth between each triangle and that it confirmed our observations about the growing rows.   

At this point I stopped all penny action and asked them to take "what you know about how they grow" and skip ahead to the 10th, 11th or 12th triangle using only the numbers to help them calculate the answer. Some kids did this easily.  Whether it was easy or less easy, most kids found it an enjoyable challenge, which I was very happy to see.

Other kids seemed less interested in solving the final challenge of skipping ahead and instead enjoyed finding patterns within the triangles they had recorded or by playing with the pennies themselves.  I consider this all good grist for the mill and great thinking for many reasons.  

If we think of learning and understanding as something that builds, one experience at a time, then I'm happy that kids are engaged in the activity in some way. If we consider that children are investigating their own ideas based in some way on the investigation I presented, then I'm all for it -- we want students to ask new questions and find new patterns.  If we always push toward a certain kind of answer we might miss out on really noticing thinking like this:

Math groups with Malke happen on Friday math game day.  One of the teachers remarked that he thought kids might not want to leave their games to come to math group. The reality has been, though, that when their group is called, they all troop willingly into my little room, often a little curious. This, coupled with their intent concentration and thoughtful work, shows me that though I may be new to teaching sit-down math (!) I am orchestrating investigations that are engaging to these eight- and nine-year old learners and flexible enough to challenge a range of math abilities. Their skills grow, my skills grow. We all enjoy math. Win-win-WIN.